Probability Calculator Craps
Craps Odds and Probabilities - Calculating Craps Combinations
This free probability calculator can calculate the probability of two events, as well as that of a normal distribution. Learn more about different types of probabilities, or explore hundreds of other calculators covering the topics of math, finance, fitness, and health, among others. Any Craps Any Seven: What is the probability of consecutive? What is the probability that exactly decisions will. Out of total decisons?
The probability of rolling any of the other numbers can be calculated in the same fashion. Once players understand how to calculate probability, they can proceed to calculate the house edge. It would be best to provide an example to make things as clear as possible. The Craps 2 bet wins whenever a total of 2 comes up on the next roll of the dice. Dice Roll Odds Understanding dice rolling probability isn't much different than a coin flip probability. A coin has two sides. If you flip a coin,probability dictates that either heads or tails have a 50% chance of coming up. If you choose heads before the flipping of a coin, then there's 1 way of 2 that would happen.
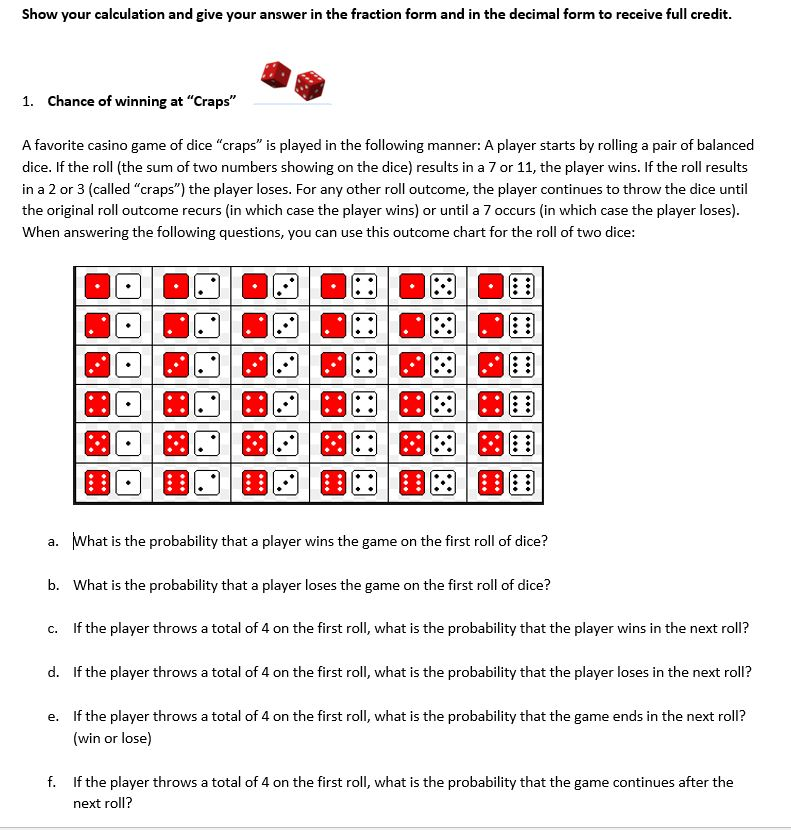
Calculating craps odds and probability seems hard, but it's not as complicated as one might think. When calculating the probabilities of any gambling activity, the first thing one looks at is the number of potential outcomes. When rolling two six-sided dice, like you in a game of craps, there are 36 possible outcomes. (There are only 11 possible totals, 2 through 12, but there are 36 combinations that can result in those totals.)
There is only one way to roll a 2 (or a 12). Roll a 1 on each die (or a 6 on each die.) Since there are 36 possible combinations, and only 1 of those combinations can total 2, the probability of getting a 2 on a roll is 1 out of 36, or 35 to 1, as stated in odds terms. There are 2 ways to roll a 3 though - you can roll a 1 and a 2, or roll a 2 and a 1, so the probability of rolling a 3 is 2 out of 36. 2 out of 36 is the same as 1 out of 18, which stated in odds terms is 17 to 1.
Here's a chart outlining the possible combinations, how many ways each total can be rolled, and what the odds are for each total.

| Total | Number of Ways to Roll This Total | Odds | Combinations |
| 2 | 1 | 35 to 1 | 1,1 |
| 3 | 2 | 17 to 1 | 1,2 + 2,1 |
| 4 | 3 | 11 to 1 | 1,3 + 3,1 + 2,2 |
| 5 | 4 | 8 to 1 | 1,4 + 4,1 + 2,3 + 3,2 |
| 6 | 5 | 6.2 to 1 | 1,5 + 5,1 + 2,4 + 4,2 + 3,3 |
| 7 | 6 | 5 to 1 | 1,6 + 6,1 +2,5 + 5,2 + 3,4 + 4,3 |
| 8 | 5 | 6.2 to 1 | 2,6 + 6,2 + 3,5 + 5,3 +4,4 |
| 9 | 4 | 8 to 1 | 3,6 + 6,3 + 4,5 +5,4 |
| 10 | 3 | 11 to 1 | 4,6 + 6,4 + 5,5 |
| 11 | 2 | 17 to 1 | 5,6 + 6,5 |
| 12 | 1 | 35 to 1 | 6,6 |
Calculating the Odds in Craps
The formula used to calculate the odds of rolling a specific total in craps is actually pretty simple. Divide 36 by the number of combinations that will make that total. Since there are 6 combinations which will total 7, the probability is 36 divide by 6, or 1 in 6 chance of rolling a 7.
Converting this to odds is easy to. Odds are always stated as the number of possibilities of something not happening versus the number of possibilities of something happening. For every roll of 7 there will be 5 rolls that aren't 7.
Craps Probabilities and the House Edge
The house edge is the difference between what the house pays out on a bet and it's true odds. For example, if a casino pays $30 for a $1 bet that someone will roll a 2, it's making a profit, because the true odds are 35 to 1. To be a break even bet, the casino would need to pay out $35 on that bet. (And return the $1 bet.) But if a casino had nothing but break even bets, it wouldn't make a profit. Without a profit, they'd have no reason to exist, and one wouldn't get to play craps.

It's good to understand how probabilities and odds are calculated because not all bets have as high a house edge as other bets. If one understands the math behind the odds, one can then choose the better bets, the ones with the lowest house edge. Choosing the bets with the lowest house edge is just good craps strategy.
Introduction
J.L.Kelly, in his seminal paper A New Interpretation of Information Rate (Bell System Technical Journal, 35, 917-926 see below) asked the interesting question: how much of my bankroll should I stake on a bet if the odds are in my favor? This is the same question that a business owner, investor, or speculator has to ask themself: what proportion of my capital should I stake on a risky venture?
Kelly did not, of course, use those precise words — the paper being written in terms of an imaginary scenario involving bookies, noisy telephone lines, and wiretaps so that it could be published by the prestigious Bell System Technical journal.
Assuming that your criterion is the same as Kelly's criterion — maximizing the long term growth rate of your fortune — the answer Kelly gives is to stake the fraction of your gambling or investment bankroll which exactly equals your advantage. The form below allows you to determine what that amount is.
Please read the disclaimer, if you haven't done so already.
Results
- The odds are in your favor, but read the following carefully:
- According to the Kelly criterion your optimal bet is about 5.71% of your capital, or $57.00.
- On 40% of similar occasions, you would expect to gain $99.75 in addition to your stake of $57.00 being returned.
- But on those occasions when you lose, you will lose your stake of $57.00.
- Your fortune will grow, on average, by about 0.28% on each bet.
- Bets have been rounded down to the nearest multiple of $1.00.
- If you do not bet exactly $57.00, you should bet less than $57.00.
- The outcome of this bet is assumed to have no relationship to any other bet you make.
- The Kelly criterion is maximally aggressive — it seeks to increase capital at the maximum rate possible. Professional gamblers typically take a less aggressive approach, and generally won't bet more than about 2.5% of their bankroll on any wager. In this case that would be $25.00.
- A common strategy (see discussion below) is to wager half the Kelly amount, which in this case would be $28.00.
- If your estimated probability of 40% is too high, you will bet too much and lose over time. Make sure you are using a conservative (low) estimate.
- Please read the disclaimer as well as the notes below.
More Information
The BJ Math site used to contain a great collection of papers on Kelly betting, including the original Kelly Bell Technical System Journal paper. Unfortunately it is now defunct, and only contains adverts for an online casino. However, you can find much of the content through the Wayback Machine archive. The Internet Archive also contains a copy of Kelly's original paper which appeared as A New Interpretation of Information Rate, Bell System Technical Journal, Vol. 35, pp917-926, July 1956. (If this link breaks — as it has done several time since this page was written — try searching for the article title).
We based the above calculations on the description given in the book Taking Chances: Winning With Probability by John Haigh, which is an excellent introduction to the mathematics of probability. (Note that there is a misprint in the formula for approximating average growth rate on p359 (2nd edition) and the approximation also assumes that your advantage is small. There is a short list of corrections which can be found through John Haigh's web page).
Probability Calculator Craps Games
Note that although the Kelly Criterion provides an upper bound on the amount that should be risked, there are sound arguments for risking less. In particular, the Kelly fraction assumes an infinitely long sequence of wagers — but in the long run we are all dead. It can be shown that a Kelly bettor has a 1/3 chance of halving a bankroll before doubling it, and that you have a 1/n chance or reducing your bankroll to 1/n at some point in the future. For comparison, a “half kelly” bettor only has a 1/9 chance of halving their bankroll before doubling it. There's an interesting discussion of this (not aimed at a mathematical reader) in Part 4 of the book Fortune's Formula which gives some of the history of the Kelly criterion, along with some of its notable successes and failures.
Probability Calculator Craps Game
Jeffrey Ma was one of the members of the MIT Blackjack Team, a team which developed a system based on the Kelly criterion, card counting, and team play to beat casinos at Blackjack. He has written an interesting book The House Advantage, which examines what he learned about managing risk from playing blackjack. (He also covers some of the measures put in place by casinos to prevent the team winning!)
See also: suggested books on probability and statistics and suggested books on investment and automated trading.
Disclaimer
The Kelly Strategy Bet Calculator is intended for interest only. We don't recommend that you gamble.We don't recommend that you place any bets based upon the results displayed here. We don't guarantee the results. Use entirely at your own risk.